31 Great Circles of the Icosahedron (FREE PNG)
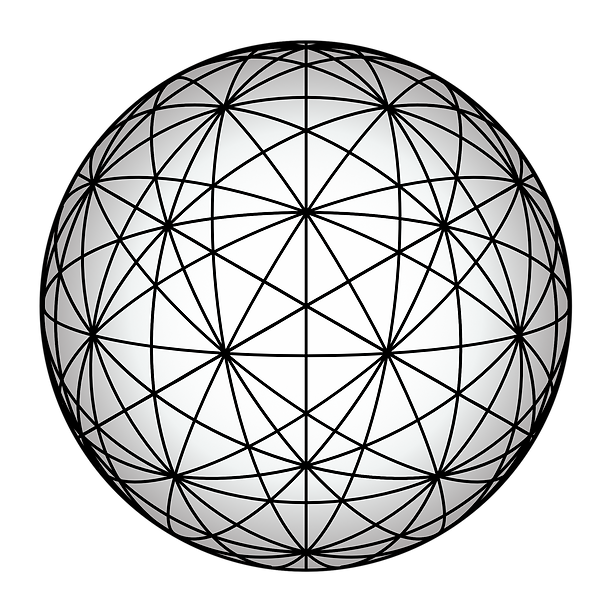
A great circle is one that shares the radius and center with the sphere in question. The equator of the earth is an example of a great circle. The tropic of Cancer and the Tropic of Capricorn are two examples of lesser circles as they do not pass through the center of the earth sphere. A great circle defines the shortest distance between two points on the surface of a sphere.
Fuller found that rotating polyhedra about their axis of symmetry generates "spin equators" (great circles) that give insight into topological accounting.
457.01 Three Sets of Axes of Spin: The icosahedron has three unique symmetric sets of axes of spin. It provides 20 triangular faces, 12 vertexes, and 30 edges. These three symmetrically interpatterned topological aspects – faces, vertexes, and mid-edges – provide three sets of axes of symmetric spin to generate the spherical icosahedron projection's grid of 31 great circles.
To read the entirety of Synergetics 1 & 2 - visit: http://rwgrayprojects.com/synergetics/synergetics.html
* THIS GRAPHIC IS IN THE PUBLIC DOMAIN AND CAN BE USED FOR ANY PURPOSE WITHOUT ATTRIBUTION (although it is appreciated)
